Authored by: Olaf Simmat, Daniel Toro, Alexander Schenk zu Schweinsberg, Andreas Vester

Determination of orbital mixing parameters for small volumes under particular consideration of the surface tension

Determination of orbital mixing parameters for small volumes under particular consideration of the surface tension

1. Overview

Surface and interfacial tension is a key factor to determine the liquid distribution in small laboratory vessels, frequently used in laboratory automation, under orbital mixing conditions. This concerns in particular vessels with a diameter smaller than 8 mm and microplates with 96 or more wells.

A practically applicable mathematical model was developed to calculate the liquid distribution in a laboratory vessel depending on operation conditions, geometry and further physical parameters with consideration of surface forces. The model enables a time efficient way to calculate optimal operation conditions, in particular mixing speed ω and orbital radius r0.

The developed computational model was examined based on experimental results of studies conducted with orbital shakers of the BioShake® series.

2. Introduction

The determination of appropriate operation parameters depends on

  • physiochemical properties of the liquid sample (density ρ, kinematic viscosity ν, surface tension σ, volume VF, temperature T)
  • surface properties of the solid vessel construction material
  • geometry of the vessel
  • technical properties of the mixing device (in particular orbital shaking amplitude r0 and mixing frequency ω).

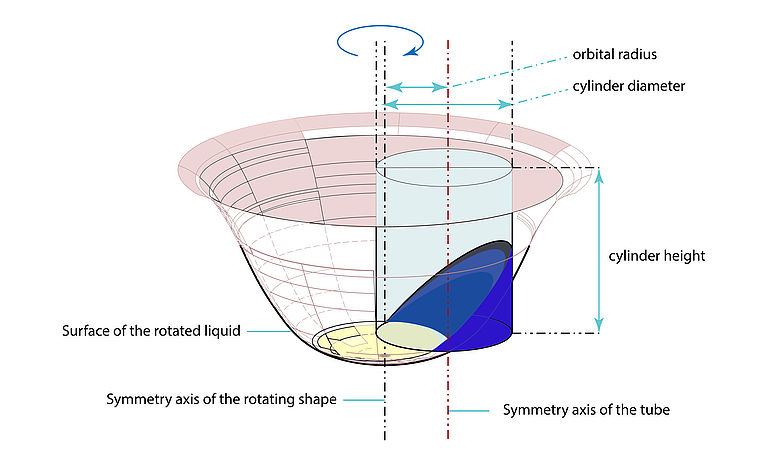
In Figure 1 we illustrate selection criteria for appropriate technical properties (r0, ω). To achieve an effective mixing process the formation of a macroscopic flow inside the vessel is the most important requirement. The orbital radius r0  has a great impact on the liquid motion and should be selected with respect to the parameters listed above.

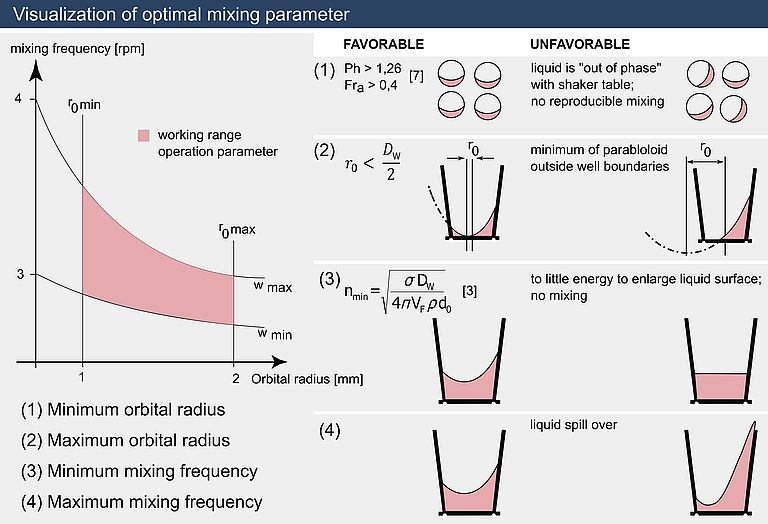
Figure 1. Correlation between orbital mixing parameters and liquid motion
Figure 1. Correlation between orbital mixing parameters and liquid motion
  1. Below a defined value r0, the liquid is not able to follow the external excitation and is “out-of-phase” with the motion of the shaker table [6, 7]. This avoids parallel and reproducible sample treatment.
     
  2. For a fast and effective mixing process r0 should be smaller than the vessel radius R. As a result the minimum of the liquid paraboloid rotates within the boundaries of the vessel and provides effective mixing, avoids separation by centrifugal forces and swirls up present particles or high density liquids.
     
  3. The minimum value for the mixing frequency ωmin is defined to overcome the resistance forces caused by fluid´s viscosity and the surface tension. Only above this value the applicated energy is high enough to enlarge the liquid surface [3].
     
  4. The mixing frequency ωmax is defined as the value were the maximum liquid height of the sample reaches the upper limit of the vessel. This could lead to spill over if no lid is present and in case of microplates to cross-contamination.

3. Mathematical Model

The free surface profile of a liquid in a vessel, rotating about its longitudinal axis, is considered as paraboloid if viscosity, surface and interfacial tensions are neglected.

Based on this assumption Buechs et. Al. [2] presented a method for calculating liquid distribution in shake flasks on rotary shakers with a good agreement to experimental data for 250 ml conical flasks. For small vessel dimensions, frequently used in laboratory automation, surface and interfacial tension have a major impact on the resulting liquid distribution [3, 4].

Figure 2. Illustration of different cases of liquid distribution based on [2].
Figure 2. Illustration of different cases of liquid distribution based on [2].

Based on equations presented by Lubarda [5] a mathematical program was developed to simulate liquid motion in small vessels under orbital mixing conditions. For the given parameters angular velocity ω, vessel radius R, surface tension σ, fill volume VF and liquid density ρ the surface profile is obtained as described below.

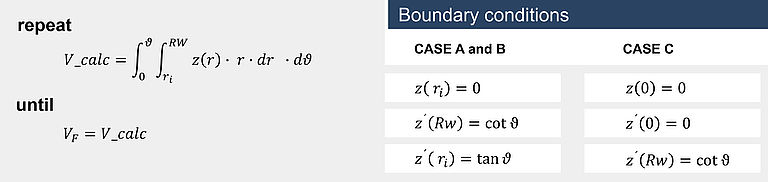
The numerically solution of the model is solved in an semi-inverse manner according to the following steps :  

  • determination of an equivalent radius Rw(θ)
  • numerical solution of the governing equations
  • calculation of an analytical rotational equation z(r)gained from the previous numerical solution
  • volume integration of the liquid, enclosed between the rotational surface z(r) and the vessel wall and bottom; the mathematical definition of φ and ri are illustrated in figure 2 and are depended on the relevant liquid distribution case
  • the liquid distribution case selection is a consequence of procedure of exclusion; it begins with case A and ends with case C
  • the procedure repeats until the boundary conditions are fulfilled.

 

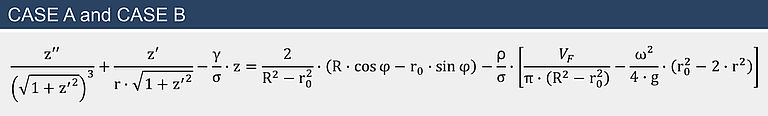
formel_case_AB_final____handout.jpg
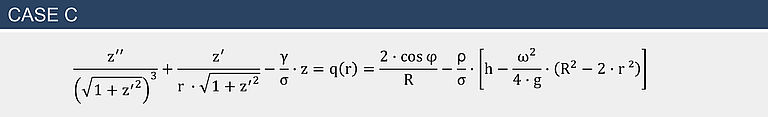
formel_case_C_final____handout.jpg
boundary_conditions_final_RepeatUntil.jpg

4. Results

In figure 3 and 4 a comparison between simulation of the free surface of the liquid sample under orbital mixing conditions using the developed model and experimental results conducted with orbital shakers of the BioShake® series are shown.

Figure 3. Liquid distribution in a 384-well microplate (ThermoScientific 95040000, Material PS, contact angle φ=87,4°), VF= 16µl Aqua dest.+E124 (300:1), r0=1mm, T=19°C
Figure 3. Liquid distribution in a 384-well microplate (ThermoScientific 95040000, Material PS, contact angle φ=87,4°), VF= 16µl Aqua dest.+E124 (300:1), r0=1mm, T=19°C
Figure 4: Liquid distribution in a 96-well microplate (Nunc 269620, material PS contact angle φ=87,4°), VF=60µl Aqua dest.+E124 (300:1), r0=1mm, T=19°C.
Figure 4: Liquid distribution in a 96-well microplate (Nunc 269620, material PS contact angle φ=87,4°), VF=60µl Aqua dest.+E124 (300:1), r0=1mm, T=19°C.

The predicted liquid distribution with consideration of surface and interfacial tension shows good concordance to the experimental data. Furthermore, it is obvious that the calculation of the liquid distribution in absence of surface tension does not show a good correlation for small laboratory vessels with a radius R smaller than 4 mm. The results do not only match with the maximum height of the profile they also correlate well with the liquid distribution profile.

In figure 5 the influence of the contact angle for different vessel construction materials and liquids is presented.

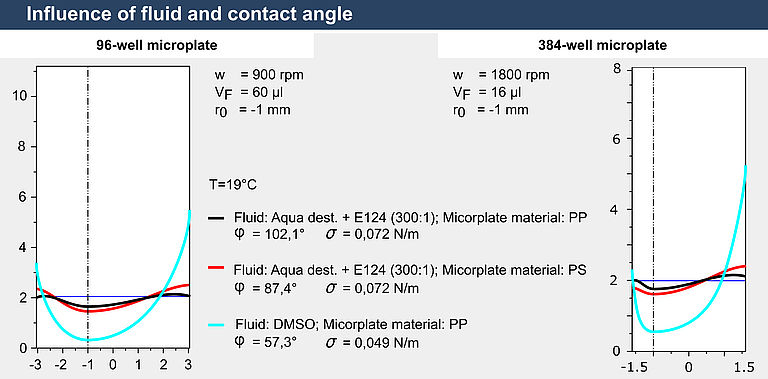
Figure 5. Example calculation for different liquid samples and construction materials under constant operation conditions.
Figure 5. Example calculation for different liquid samples and construction materials under constant operation conditions.

The different vessel materials as well as the liquids are leading to different liquid profiles that are independent from the operation conditions (r0, ω).

As can be seen different surface tensions, due to the type of fluid or a change of the sample temperature, have a significant impact on  the liquid profile. An increased surface tension value results in a lower profile. For different fluids (Aqua dest. and DMSO) with identical filling volumes and operation conditions the maximum fluid height can differs more than 200%.

On the other side for identical liquid properties and filling volumes, the change of the contact angle due to different construction materials, which are commonly used for microplates (PS and PP), leads only to a change of less than 10% of the maximum fluid height.

5. Conclusion

Appropriate operation parameters for orbital mixing are commonly selected based on empirical experiments, which are time and cost-intensive. Wrong decisions could influence the results of experiments and cause additional costs and problems.

The desired liquid distribution in the vessel depends on the intended process. For bioreactor systems and cultivation processes the aim is to achieve a large liquid-gas interface area to maximize the gas transfer; case A ─ most liquid circulatesalongthe wall.

In contrast, for a fast and effective mixing process the orbital amplitude should be smaller than the vessel radius r0<Rand the mixing frequency high enough to get the liquid minimum at r0 as near as possible to the bottom before the liquid spills over the well boundaries; case B or C.

The determination of the free surface profile of a liquid sample in small laboratory vessels, with a radius R<4 mm, under orbital mixing conditions, in the presence of gravity and surface tension, is difficult. It requires the numerical integration of a nonlinear second-order differential equation.

We developed a program based on a mathematical approach that takes the three above-mentioned scenarios of liquid distribution into account.

The developed mathematical approach is a highly useful tool for the expert, allowing an efficient and fast simulation for various types of liquid samples, vessel geometries, construction materials, operation conditions and further parameters. The calculated results show a good concordance to the results of experimental studies.

References

[1]  SIGLOCH, H.: Technische Fluidmechanik. 1. Auflage. Springer Verlag, 2008

[2]  BÜCHS, J. ET AL.: Time efficient way to calculate Oxygen transfer Areas and power input in cylindrical disposable shaken   bioreactors. In: Biotechnology progress. 30 (2014), No. 6, pp.1441-1456

[3]  HERRMANN, R. ET AL: Characterisation of Gas-Liquid Mass Transfer Phenomena in  Microplates.
      In: Biotechnology and Bioengineering 81 (2003), No. 2, pp. 178-186

[4]  DUETZ, W.A.: Mictotiter plates as mini-bioreactors: miniaturization of fermentation methods.
      In: TRENDS in Microbiology 15 (2007), No. 10, pp. 469-475

[5]   LUBARDA, V.A. : The shape of a liquid surface in a uniformly rotating cylinder in the presence of surface tension.

  In: Acta Mechanica 224 (2013) No.7, pp.1365-1382

[6]  HANG, H. ET AL: Engineering characterisation of a single well from 24-well and 96-well microtitre plates.
      In: Biochem. Eng. J. (2008), No.40, pp.138-149

[7]  BÜCHS, J. ET AL.: Out-of-phase operating conditions, a hithero unknown phenomenon in shaking bioreactors.
      In: Biochemical Engineering Journal (2001), No. 7, pp. 135-141

[8]  Klöckner, W. ET AL.: Advances in shaking technologies. In: Trends Biotechnol. (2012), No. 30, pp.307–314

Downloads

Influence of the surface tensionDetermination of orbital mixing parameters for small volumes under particular consideration of the surface tension4 MB

Audio

[AUDIO] Determination of orbital mixing parameters for small volumes under particular consideration of the surface tension